by Johan Hoffman and Claes Johnson

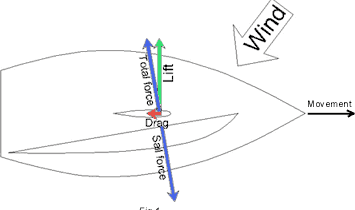
This article solves the incompressible Navier-Stokes equations with friction force boundary conditions and shows that the classical inviscid circulation theory by Kutta-Zhukovsky for lift and laminar viscous boundary layer theory by Prandtl for drag, which have dominated 20th century fluid dynamics, do not correctly describe the real turbulent airflow around a sail under tacking. We show that lift and drag essentially originate from a turbulent wake of counter-rotating rolls of low-pressure streamwise vorticity generated by a certain instability mechanism of potential flow at rear separation. The new theory opens the possibility of ab initio computational prediction of characteristics of a sailing boat using les than a million meshpoints without resolving thin boundary layers, instead of the imposssible quadrillions required according to state-of-the-art for boundary layer resolution.

Read the whole article here